Control de vibración de estructuras excitadas sísmicamente usando un dispositivo con sistema inerter
Vibration control of seismicly excited structures using a device with inerter system
Barra lateral del artículo
PDF
FLIP
Términos de la licencia (VER)
Esta obra está bajo una licencia internacional Creative Commons Atribución-NoComercial-SinDerivadas 4.0.
Declaración del copyright
Los autores ceden en exclusiva a la Universidad EIA, con facultad de cesión a terceros, todos los derechos de explotación que deriven de los trabajos que sean aceptados para su publicación en la Revista EIA, así como en cualquier producto derivados de la misma y, en particular, los de reproducción, distribución, comunicación pública (incluida la puesta a disposición interactiva) y transformación (incluidas la adaptación, la modificación y, en su caso, la traducción), para todas las modalidades de explotación (a título enunciativo y no limitativo: en formato papel, electrónico, on-line, soporte informático o audiovisual, así como en cualquier otro formato, incluso con finalidad promocional o publicitaria y/o para la realización de productos derivados), para un ámbito territorial mundial y para toda la duración legal de los derechos prevista en el vigente texto difundido de la Ley de Propiedad Intelectual. Esta cesión la realizarán los autores sin derecho a ningún tipo de remuneración o indemnización.
La autorización conferida a la Revista EIA estará vigente a partir de la fecha en que se incluye en el volumen y número respectivo en el Sistema Open Journal Systems de la Revista EIA, así como en las diferentes bases e índices de datos en que se encuentra indexada la publicación.
Todos los contenidos de la Revista EIA, están publicados bajo la Licencia Creative Commons Atribución-NoComercial-NoDerivativa 4.0 Internacional
Licencia
Esta obra está bajo una Licencia Creative Commons Atribución-NoComercial-NoDerivativa 4.0 Internacional
Contenido principal del artículo
Resumen
Una parte importante en el proceso de diseño estructural es disminuir el efecto
de vibración en edificios, particularmente en aquellos que pueden estar sujetos a
excitación sísmica. Aunque investigaciones en el campo del control estructural se
han venido desarrollando desde principios de 1900, la complejidad y dimensiones
de las estructuras civiles hacen que este problema sea particularmente difícil
de resolver debido a la gran demanda de esfuerzo de control y muchas otras
limitaciones asociadas. Este artículo analiza el rendimiento de un sistema de
control pasivo novedoso que puede ser utilizado para el control de vibraciones en
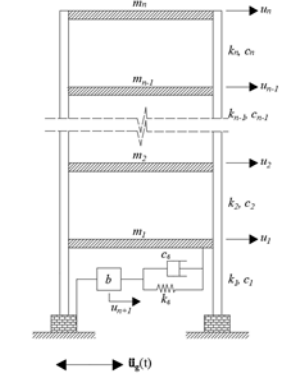
estructuras civiles sometidas a excitaciones en la base. Este dispositivo de control
es denominado amortiguador sintonizado inerter (Tuned Inerter Damper, TID)
el cual supera la limitación de los dispositivos pasivos clásicos que son eficientes
en una banda de frecuencia estrecha para la cual están sintonizados, situación
que resulta inconveniente debido a que los terremotos muestran un contenido
frecuencial diverso en la mayoría de ocasiones. Este estudio emplea, además,
un enfoque de optimización metaheurística basado en el método de evolución
diferencial (ED), combinado con un análisis dinámico tiempo-historia elástico,
a través del cual el control de vibración se enfoca en dos objetivos individuales:
primero, minimizar el desplazamiento máximo horizontal; y segundo, minimizar la
media cuadrática de desplazamiento (Root Mean Square, RMS). Se estudia el caso
de un edificio de 12 pisos equipado con una ubicación novedosa del TID sometido
a múltiples excitaciones sísmicas para verificar la efectividad del dispositivo y
diferente a la presentada en la literatura. Los resultados muestran una mejora
significativa en la respuesta dinámica cuando se emplea la disposición presentada
en este trabajo y considerablemente mejor que un TMD equivalente. Por lo tanto, el TID representa una alternativa potencialmente atractiva de las técnicas tradicionales de control pasivo.
Descargas
Detalles del artículo
Referencias (VER)
Pan, C., & Zhang, R. (2018). Design of structure with inerter system based on stochastic response mitigation ratio. Structural Control and Health Monitoring, 25(6). doi:https://doi.org/10.1002/stc.2169
Bekdaş, G., & Nigdeli, S. M. (2011). Estimating optimum parameters of tuned mass dampers using harmony search. Engineering Structures, 33(9), 2716-2723. doi:http://dx.doi.org/10.1016/j.engstruct.2011.05.024
BekdaşG, Nigdeli, S. M., & Yang, X. S. (2018). A novel bat algorithm based optimum tuning of mass dampers for improving the seismic safety of structures. Engineering Structures, 159, 89-98. doi:http://dx.doi.org/10.1016/j.engstruct.2017.12.037
Biswas Raha, S., & Chakraborty, N. (2012). Tuned reactive power dispatch through modified differential evolution technique. Frontiers in Energy, 6, 138-147. doi:http://dx.doi.org/10.1007/s11708-012-0188-8
Buckle, G. (2000). Passive control of structures for seismic loads. Bull. N.Z. Natl. Soc. Earthq. Eng, 33(3), 209-221. doi:https://doi.org/10.5459/bnzsee.33.3.209-221.
Bureerat, S., & Pholdee, N. (2017). Adaptive sine cosine algorithm integrated with differential evolution for structural damage detection. Computational Science and Its Applications–ICCSA 2017: 17th International Conference, 71-86. doi:http://dx.doi.org/10.1007/978-3-319-62392-4_6
Chen, Y. C., Tu, J. Y., & Wang, F. V. (2015). Earthquake vibration control for buildings with inerter networks. 2015 European Control Conference (ECC), 3137-3142. doi:http://dx.doi.org/10.1109/ECC.2015.7331016
Den Hartog, J. P. (1985). Mechanical vibrations. New York: Courier Corporation.
Farshidianfar, A., & Soheili, S. (2013). Optimization of TMD parameters for earthquake vibrations of tall buildings including soil structure interaction. Iran University of Science & Technology, 3(3), 409-429.
Frahm, H. (1911). Device for damping vibrations of bodies. U.S. Pat. No 989,958, 1911. doi:https://doi.org/10.1016/j.tree.2005.10.010
Hu, Y., & Chen, M. Z. (2015). Performance evaluation for inerter-based dynamic vibration absorbers. Int. J. Mech. Sci, 99, 297-307. doi:https://doi.org/10.1016/j.ijmecsci.2015.06.003
Inc, T. M. (2019). MATLAB R2019a. MA, USA: Natick.
Kelly, J. M. (1998). Base isolation: Origins and development. EERC News, 12(1).
Kelly, J. M., & Beucke, K. E. (1983). A frictional damped base isolation system with fail-safe characteristics. Earthq. Eng. Struct. Dynam, 11, 33-56.
Kelly, J. M., & Hodder, S. B. (1982). Experimental study of lead and elastomeric dampers for base isolation system in laminated neoprene bearings. Bull. N.Z. Nat. Soc. Earthq. Eng, 15, 53-67.
Lazar, I. F., Neild, S. A., & Wagg, D. J. (2014). Design and performance analysis of inerter-based vibration control systems. Dynamics of Civil Structures, 4, 493-500. doi:http://dx.doi.org/10.1007/978-3-319-04546-7_53
Lazar, I. F., Neild, S. A., & Wagg, D. J. (2014). Using an inerter‐based device for structural vibration suppression. Earthq. Eng. Struct. Dyn, 43(8), 1129-1147. doi:https://doi.org/10.1002/eqe.2390
Lazar, I. F., Wagg, D. J., & Neild, S. A. (2013). An inerter vibration isolation system for the control of seismically excited structures. 10th International Conference on Urban Earthquake Engineering.
Lazar, I., Neild, S., & Wagg, D. (2014). Inerter-based Vibration Suppression Systems for Laterally and Base-Excited Structures. (E. C. A. Cunha, Ed.) Proceedings of the 9th International Conference on Structural Dynamics.
Leung, A. Y., & Zhang, H. (2009). Particle swarm optimization of tuned mass dampers. Engineering Structures, 31(3), 715-728. doi:http://dx.doi.org/10.1016/j.engstruct.2008.11.017
Ministerio de Vivienda, C. y. (2010). Reglamento Colombiano de Construcción Sismo Resistente NSR-10. Bogotá: AIS.
Nigdeli, S. M., Bekdas, G., & Yang, X. S. (2016). Optimum tuning of mass dampers for seismic structures using flower pollination algorithm. Int. J. Theor. Appl. Mech, 1, 264-268.
Ormondroyd, J., & Den Hartong, J. P. (1928). The theory of the dynamic vibration absorber. Transaction of the ASME, 50, 9-22.
Papageorgiou, C., & Smith, M. (2005). Laboratory experimental testing of inerters. Proceedings of the 44th IEEE Conference on Decision and Control, and the European Control Conference. doi:https://doi.org/10.1109/CDC.2005.1582679
Said, E. (2018). Seismic Energy Assessment of Buildings with Tuned Vibration Absorbers. Shock and Vibration, 2018, 1-10. doi:https://doi.org/10.1155/2018/2051687
Seyedpoor, S. M., Shahbandeh, S., & Yazdanpanah, O. (2015). An efficient method for structural damage detection using a differential evolution algorithm-based optimisation approach. Civil Engineering and Environmental Systems, 32(3), 230-250. doi:http://dx.doi.org/10.1080/10286608.2015.1046051
Shen, W., Niyitangamahoro, A., Feng, Z., & Zhu, H. (2019). Tuned Inerter Dampers for Civil Structures Subjected to Earthquake Ground Motions: optimum design and seismic performance. Engineering Structures, 198. doi:https://doi.org/10.1016/j.engstruct.2019.109470
Shen, Y., Chen, L., Yang, X., Shi, D., & Yang, J. (2016). Improved design of dynamic vibration absorber by using the inerter and its application in vehicle suspension. Journal of Sound and Vibration(361), 148-158. doi:http://dx.doi.org/10.1016/j.jsv.2015.06.045
Smith, M. (2002). Synthesis of mechanical networks: The inerter. IEEE Transactions on automatic control, 47(10), 1648-1662. doi:https://doi.org/10.1109/TAC.2002.803532
Storn, R., & Price, K. (1997). Differential Evolution - A Simple and Efficient Heuristic for Global Optimization over Continuous Spaces. J. Global Optim, 11(4), 341-359. doi:https://doi.org/10.1023/A:1008202821328.
Vo-Duy, T., Ho-Huu, V., Dang-Trung, H., & Nguyen-Thoi, T. (2016). A two-step approach for damage detection in laminated composite structures using modal strain energy method and an improved differential evolution algorithm. Composite Structures, 147, 42-53. doi:http://dx.doi.org/10.1016/j.compstruct.2016.03.027
Wang, F. C., Chen, C. W., Liao, M. K., & Hong, M. F. (2007). Performance analyses of building suspension control with inerters. 46th IEEE Conference on Decision and Control, 3786-3791.
Wang, F. C., Hong, M. F., & Chen, C. W. (2010). Building suspensions with inerters. Proceedings of the Institution of Mechanical Engineers, Part C. J. Mech. Eng. Sci, 224(8), 1605-1616. doi:https://doi.org/10.1243/09544062JMES1909.
Wen, Y., Chen, Z., & Hua, X. (2017). Design and evaluation of tuned inerter-based dampers for the seismic control of MDOF structures. Journal of Structural Engineering, 143(4). doi:http://dx.doi.org/10.1061/(ASCE)ST.1943-541X.0001680
Yucel, M., Bekdaş, G., Nigdeli, S. M., & Sevgen, S. (2019). Estimation of optimum tuned mass damper parameters via machine learning. Journal of Building Engineering, 26.